Physics Keypoints: Equilibrium of Forces;In the realm of physics and engineering, the concept of equilibrium plays a pivotal role in explaining how objects remain stable and motionless under the influence of various forces. In this comprehensive explanation, we will delve into the fundamental aspects of equilibrium of forces, covering a wide array of topics to provide a thorough understanding.
Read other physics keypoints here
Physics Keypoints: Equilibrium of Forces
I. Equilibrium of Particles:
(a) Equilibrium of Coplanar Forces:
When multiple forces act on a single particle, it is said to be in equilibrium if the vector sum of all these forces equals zero. This means that the forces are balanced and do not result in any net movement. Coplanar forces refer to forces lying in the same plane.
Illustration: Consider a book resting on a table. The gravitational force pulling it downward is balanced by the normal force exerted by the table, resulting in equilibrium.
(b) Triangles and Polygon of Forces:
Triangles and polygons of forces are graphical techniques used to determine the resultant of multiple forces. The triangle method involves representing forces as sides of a triangle and closing the triangle to find the resultant. In the polygon method, forces are represented as sides of a polygon, and the resultant is determined by closing the polygon.
Example: If three forces are acting on an object, the triangle or polygon methods can be employed to determine the resultant force and its direction.
(c) Lami’s Theorem:
Lami’s theorem is particularly useful when three concurrent forces are involved. It asserts that for an object to remain in equilibrium under the influence of three forces, each force must be proportional to the sine of the angle between the other two forces. This theorem is valuable in structural analysis and design.
II. Principles of Moments:
(a) Moment of a Force:
The moment of a force about a point is a measure of its turning effect or torque. It is calculated by multiplying the force magnitude by the perpendicular distance from the point to the line of action of the force. The moment of a force is essential in understanding rotational motion and equilibrium.
Application: When opening a door, the turning force applied to the doorknob generates a moment that causes the door to swing open.
(b) Simple Treatment and Moment of a Couple (Torque):
A couple is a pair of equal and opposite forces acting in parallel but not along the same line. The moment of a couple is the product of one of the forces and the distance between them. Couples are vital in rotational dynamics and engineering applications.
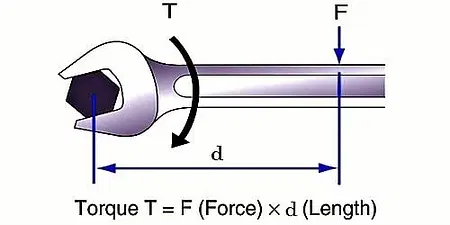
Illustration: A wrench applying torque to a bolt is an example of a couple in action.
(c) Applications:
Moments are ubiquitous in engineering and physics. They find applications in designing lever systems, analyzing the stability of structures, and understanding how forces induce rotation in machines and objects.
III. Conditions for Equilibrium of Rigid Bodies:
(a) Resolution and Composition of Forces in Two Perpendicular Directions:
For a rigid body to remain in equilibrium, it must satisfy the equilibrium conditions in both the horizontal and vertical directions. This entails breaking down forces into their components along these axes and ensuring that the resultant forces in each direction sum to zero.
Example: When designing a bridge, engineers must ensure that the forces acting on the structure cancel each other out horizontally and vertically for it to remain stable.
(b) Resultant and Equilibrant:
The resultant force of a set of forces is the single force that can replace them without altering their effect. Conversely, the equilibrant is the force required to balance a given set of forces, resulting in a state of equilibrium.
IV. Centre of Gravity and Stability:
(a) Stable, Unstable, and Neutral Equilibrium:
The center of gravity (CG) is the point where the entire weight of an object can be considered to act. In a stable equilibrium, the CG lies below the support point, ensuring that the object returns to its original position when displaced. In an unstable equilibrium, the CG is above the support point, causing the object to topple when disturbed. Neutral equilibrium occurs when the CG aligns with the support point, resulting in no net effect upon displacement.
Real-world Application: In automotive design, ensuring that a car’s CG is low helps maintain stability and prevents rollovers during turns.
In Conclusion:
A profound comprehension of the equilibrium of forces is foundational in the fields of physics and engineering. It allows us to analyze and design structures, machines, and systems that remain stable, balanced, and functional under the influence of various forces.
These principles underpin the safe and efficient functioning of countless everyday objects and structures, from bridges to vehicles and beyond.












